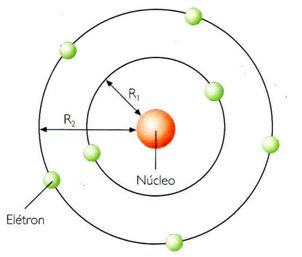
Bohr Atomic Model :

Lived 1885 - 1962. Chocolatey anydesk. Niels Bohr completely transformed our view of the atom and of the world. Realizing that classical physics fails catastrophically when things are atom-sized or smaller, he remodeled the atom so electrons occupied 'allowed' orbits around the nucleus while all other orbits were forbidden. In atomic physics, the Bohr model or Rutherford–Bohr model, presented by Niels Bohr and Ernest Rutherford in 1913, is a system consisting of a small, dense nucleus surrounded by orbiting electrons—similar to the structure of the Solar System, but with attraction provided by electrostatic forces in place of gravity.
In 1913 Bohr proposed his quantized shell model of the atom to explain howelectrons can have stable orbits around the nucleus. The motion of theelectrons in the Rutherford model was unstable because, according toclassical mechanics and electromagnetic theory, any charged particlemoving on a curved path emits electromagnetic radiation; thus, theelectrons would lose energy and spiral into the nucleus. To remedy thestability problem, Bohr modified the Rutherford model by requiring thatthe electrons move in orbits of fixed size and energy. The energy of anelectron depends on the size of the orbit and is lower for smaller orbits.Radiation can occur only when the electron jumps from one orbit toanother. The atom will be completely stable in the state with the smallestorbit, since there is no orbit of lower energy into which the electron canjump.
Bohr's starting point was to realize that classical mechanics by itselfcould never explain the atom's stability. A stable atom has a certain sizeso that any equation describing it must contain some fundamental constantor combination of constants with a dimension of length. The classicalfundamental constants--namely, the charges and the masses of the electronand the nucleus--cannot be combined to make a length. Bohr noticed,however, that the quantum constant formulated by the German physicist MaxPlanck has dimensions which, when combined with the mass and charge of theelectron, produce a measure of length. Numerically, the measure is closeto the known size of atoms. This encouraged Bohr to use Planck's constantin searching for a theory of the atom.

Bohr Atomic Number

Planck had introduced his constant in 1900 in a formula explaining thelight radiation emitted from heated bodies. According to classical theory,comparable amounts of light energy should be produced at all frequencies.This is not only contrary to observation but also implies the absurdresult that the total energy radiated by a heated body should be infinite.Planck postulated that energy can only be emitted or absorbed in discreteamounts, which he called quanta (the Latin word for 'how much'). Theenergy quantum is related to the frequency of the light by a newfundamental constant, h. When a body is heated, its radiant energy in aparticular frequency range is, according to classical theory, proportionalto the temperature of the body. With Planck's hypothesis, however, theradiation can occur only in quantum amounts of energy. If the radiantenergy is less than the quantum of energy, the amount of light in thatfrequency range will be reduced. Planck's formula correctly describesradiation from heated bodies. Planck's constant has the dimensions ofaction, which may be expressed as units of energy multiplied by time,units of momentum multiplied by length, or units of angular momentum. Forexample, Planck's constant can be written as h = 6.6x10-34 jouleseconds.
Using Planck's constant, Bohr obtained an accurate formula for the energylevels of the hydrogen atom. He postulated that the angular momentum ofthe electron is quantized--i.e., it can have only discrete values. Heassumed that otherwise electrons obey the laws of classical mechanics bytraveling around the nucleus in circular orbits. Because of thequantization, the electron orbits have fixed sizes and energies. Theorbits are labeled by an integer, the quantum number n.
With his model, Bohr explained how electrons could jump from one orbit toanother only by emitting or absorbing energy in fixed quanta. For example,if an electron jumps one orbit closer to the nucleus, it must emit energyequal to the difference of the energies of the two orbits. Conversely,when the electron jumps to a larger orbit, it must absorb a quantum oflight equal in energy to the difference in orbits.
Bohr Atomic Model Year
Excerpt from the Encyclopedia Britannica without permission.

